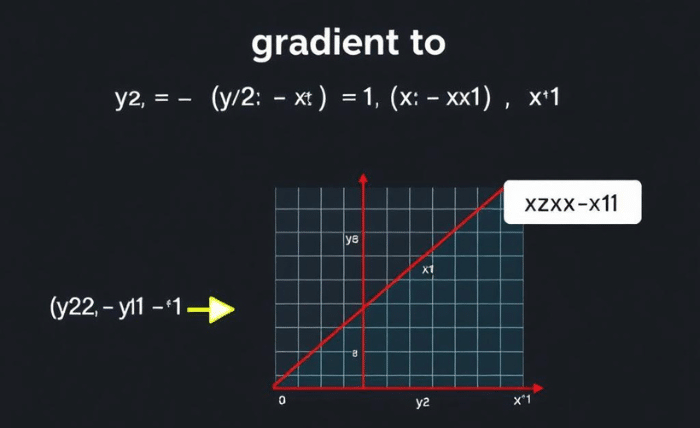
Mathematics can often seem tricky, especially when you are learning about concepts like gradients and equations. However, once you break down these ideas into simple steps, they become much easier to understand. In this blog post, we are going to explore the question: What is the gradient of the blue line Mathswatch? We’ll also take a look at how to find the equation of this blue line and why it’s so important in basic algebra and geometry.
What is the Gradient of the Blue Line Mathswatch?
When you see a graph in Mathswatch with a blue line, you may wonder how to describe its steepness or slant. That’s where the concept of gradient comes into play. Simply put, the gradient of a line tells you how steep the line is and in which direction it moves. It is a measure of how much the y-value (vertical) increases or decreases when the x-value (horizontal) increases by a certain amount.
In the Mathswatch platform, the blue line is often used in various exercises to help students understand this concept. The gradient of the blue line is a number that tells you how steep or flat the line is.
How Do You Find the Gradient of the Blue Line Mathswatch?
To understand the gradient, we need to break down how to calculate it. The gradient of a straight line is given by the formula:
Gradient=Change in yChange in x=y2−y1x2−x1\text{Gradient} = \frac{\text{Change in y}}{\text{Change in x}} = \frac{y_2 – y_1}{x_2 – x_1}Gradient=Change in xChange in y=x2−x1y2−y1
This formula is often written as:
- y2y_2y2 and y1y_1y1 are the y-coordinates of two points on the line.
- x2x_2x2 and x1x_1x1 are the x-coordinates of the same two points.
So, in simple terms, the gradient is found by calculating how much the line rises or falls (the change in y) for every unit it moves horizontally (the change in x).
What is the Equation of the Blue Line Mathswatch?
Once you know the gradient of the blue line, you can use it to find the equation of the line. The equation of a straight line is typically written as:y=mx+cy = mx + cy=mx+c
Where:
- mmm is the gradient of the line.
- ccc is the y-intercept, the point where the line crosses the y-axis.
In Mathswatch, you can often be given the gradient and a point on the line. Using this information, you can easily calculate the full equation of the line.
Step-by-Step Process to Find the Equation of the Blue Line
- Find the gradient (m): This is usually provided or you can calculate it as explained earlier using two points on the line.
- Find the y-intercept (c): This is the point where the line crosses the y-axis. If you are given a point on the line, substitute the coordinates into the equation y=mx+cy = mx + cy=mx+c to solve for ccc.
- Write the equation: Once you have the gradient and y-intercept, you can write the equation of the line in the form y=mx+cy = mx + cy=mx+c.
Why is Understanding the Gradient of the Blue Line Mathswatch Important?
Understanding the gradient is very important for several reasons:
- Slope and direction: The gradient helps you understand how steep the line is. If the gradient is positive, the line goes up as you move from left to right. If it’s negative, the line goes down.
- Real-life applications: Gradients are used in real-life situations, like when planning roads or ramps. For example, if you were building a wheelchair ramp, the gradient would tell you how steep the ramp should be.
- Graphing: Knowing how to find the gradient allows you to draw accurate graphs. This skill is essential in subjects like algebra and calculus.
What is the Gradient of the Blue Line Mathswatch: Simple Example
Let’s take an example to explain this further. Imagine you are looking at the blue line on Mathswatch, and you are given two points on the line: Point A (2, 3) and Point B (4, 7).
To find the gradient of the blue line:
- The change in y (y2−y1y_2 – y_1y2−y1) = 7 – 3 = 4
- The change in x (x2−x1x_2 – x_1x2−x1) = 4 – 2 = 2
So, the gradient of the blue line is:Gradient=42=2\text{Gradient} = \frac{4}{2} = 2Gradient=24=2
This means that for every 2 units the line moves horizontally (along the x-axis), it moves 4 units vertically (along the y-axis).
Common Mistakes to Avoid When Calculating Gradients
When working with gradients, students often make a few common mistakes. Here are some tips to help you avoid them:
- Don’t swap the coordinates: Make sure you subtract the y-values in the correct order: y2−y1y_2 – y_1y2−y1 and x2−x1x_2 – x_1x2−x1.
- Be careful with negative gradients: If the line goes down as it moves to the right, the gradient will be negative.
- Double-check your points: Always ensure that the two points you are using are correctly identified from the graph or problem.
How to Use the Gradient of the Blue Line in Mathswatch Exercises
Mathswatch is a useful tool for practicing gradient calculations. It often provides graphs where you need to identify points and calculate the gradient. Here’s how you can use Mathswatch effectively:
- Look for two points on the line: These will usually be clearly marked.
- Use the gradient formula: Apply the formula to calculate the gradient.
- Enter your answer: In some exercises, Mathswatch will ask you to enter the gradient or the equation of the line. Double-check your work before submitting.
Further Practice with the Gradient of the Blue Line Mathswatch
If you want to get better at finding the gradient and equation of the blue line on Mathswatch, practice is key. Here are some tips to help you practice:
- Work with multiple lines: Try calculating gradients for lines with different slopes (both positive and negative).
- Use graphs: Practice by plotting your own lines on graph paper and calculating the gradient.
- Watch tutorial videos: Mathswatch often provides video tutorials that can help you understand the concept more clearly.
Conclusion: What is the Gradient of the Blue Line Mathswatch?
In this blog post, we’ve explored the concept of the gradient of the blue line in Mathswatch. We’ve learned how to calculate it, how it relates to the equation of the line, and why it’s important for both understanding graphs and solving real-world problems.
By following the steps we outlined, you should now feel more confident in answering the question: What is the gradient of the blue line Mathswatch? Whether you’re practicing for exams or just improving your math skills, understanding gradients is a crucial part of learning mathematics.